Howard Wainer

“The sciences do not try to explain, they hardly even try to interpret, they mainly make models. By a model is meant a mathematical construct which, with the addition of certain verbal interpretations, describes observed phenomena. The justification of such a mathematical construct is solely and precisely that it is expected to work.”
~ John Von Neumann

Exponential growth has befuddled human intuition for centuries. Even today, with sophisticated tools and frequent contact with phenomena that exhibit exponential growth, our intuition still misleads us.

Examples of confusion engendered by the interaction of human intuition and exponential growth surround us. In 1869, Galton mis-estimated how quickly the exponential nature of the normal curve forced it to fall toward zero and hence he projected that there would be several Englishmen taller than nine feet. In Galton’s time, a nine-footer would have been about 13 standard deviations above the mean—an event of truly tiny likelihood. Without doing the calculations, you can check your own intuition by estimating the height of the normal curve at z = 0 if the height 13 standard deviations away is but one millimeter. If the metric you estimated the height in is anything smaller than the light year, your intuition is likely as flawed as was Galton’s¹.

Compound interest yields exponential growth, which is why financial planners emphasize the importance of initiating retirement savings as early in one’s life as possible. And yet it is hard to generate any strong sense, deep in one’s soul, of the effects of the exponential growth yielded by compound interest. To aid our intuition, a variety of rules of thumb have been developed. One of the best known, as well as the oldest, is the “Rule of 72” described in detail (although without derivation) by Luca Pacioli (1445–1514) in 1494.

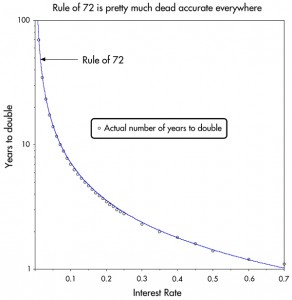
In brief, the rule of 72 allows you to calculate a good approximation to how long it will take for your money to double at any compound interest rate. The doubling time is derived by dividing the interest rate into 72. So at 6% your money will double in 12 years, at 9% in 8 years, etc.² Although this is only an easy-to-compute-in-your-head approximation, it is pretty accurate (see Figure 1).

But exponential growth happens in many other circumstances outside of finance. When I was a graduate student, John Tukey advised me that to succeed in my career I would have to work harder than my competitors, but “not a lot harder, for if you work just 10% harder in just seven years you will know twice as much as they do.” Stated in that way, it seems that at a cost of just 48 minutes a day, you can garner huge dividends.

Now that our view of the breadth of application of the Rule of 72 has been widened, we can easily see other avenues where it can provide clarity. For example, I recently attended a 50th high-school reunion and was dismayed at the state of my fellow graduates. But, once I realized that those who had allowed their weight to creep upward at even the modest rate of 1.44% a year, would, at the 50th reunion, be double the size I remembered from their
senior portrait.

In the same way, if we can increase automobile gas mileage just 4% each year, in just 18 years, the typical new car would use half the gasoline as today’s cars.

Of course, this rule also provides insight into how effective various kinds of plans for world doimination can be instituted. One possible way for a culture to dominate all others is for its population to grow faster than its competitors. But not a lot faster; for again, if the growth rate is just 12% greater, it will grow twice as much in just six years.

Here, I join with Mark Twain in that what we both like best about science is that “one gets such wholesale returns of conjecture out of such a trifling investment of fact.”

¹The height at z = 0 is approximately 3.4 million times the diameter of the universe.

²The Rule of 72 is only an approximation, the exact equation describing a doubling in T time periods for an interest rate r is T= ln(2)/r; for tripling it is T= ln(3)/r , etc. Note that 100xLn(2) = 69.3, which would provide a slightly more accrate estimate than 72; but because 72 has so many more integer factors it is easy to see why it has been preferred.

Further Reading

Pacioli, L. 1494. Summa de arithmetica. Venice, Folio 181, Page 44.

Slavin, S. 1989. All the math you’ll ever need. John Wiley & Sons. pp. 153–154.

Twain, M. 1883. Life on the Mississippi. Montreal: Dawson Brothers.

Wainer, H. 2007. Galton’s normal is too platykurtic. CHANCE 20(2):57–58.

Wainer, H. 2009. Picturing the uncertain world: How to understand, communicate, and control uncertainty through graphical display (Chapter 16). Princeton, NJ: Princeton University Press.

About the Author

Howard Wainer is distinguished research scientist at the National Board of Medical Examiners and professor of statistics at the Wharton School, University of Pennsylvania. He has won numerous awards and is a Fellow of the American Statistical Association and the American Educational Research Association. His interests include the use of graphical methods for data analysis and communication, robust statistical methodology, and the development and application of generalizations of item response theory. He has published many books; his latest, written with Lawrence Hubert, is A Statistical Guide for the Ethically Perplexed.